Description

| Material | Modulus of elasticity | ||
|---|---|---|---|
| Poisson’s ratio | |||
| Geometry | Cross-section | Tube | |
| Boundary conditions | In point A | Fixed support | |
| Loads | In point B |
Note: the arc is approximated with 16 line segments
Results

Horizontal deformation ![]() in Diamonds
in Diamonds
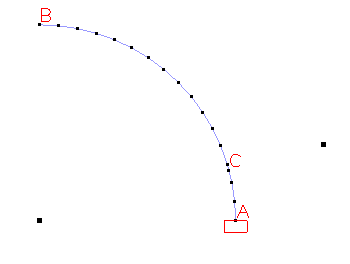
| Point | Which result | Independent reference | Diamonds | Difference |
|---|---|---|---|---|
| B | Horizontal deformation |
134,62mm | 132,03mm | -1,92% |
| C ( |
Torsional moment |
74,118 Nm | 75,582Nm | 1,98% |
| C ( |
Bending moment |
-96,593 Nm | -95,369Nm | -1.27% |
References
- Mécaniciens, S. F. D. (1990). Guide de validation des progiciels de calcul des structures: SSLL 07: arc mince encastré en flexion hors du plan.
- Tested in Diamonds 2023r01.