| Soil is a mixture of soil grains, water and air. The air is the space between the soil grains. | 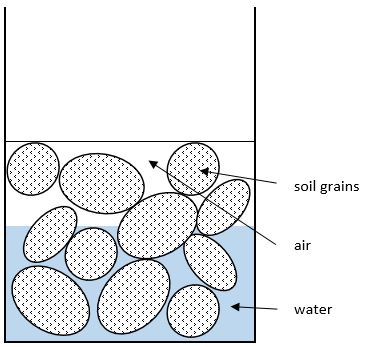 |
| When soil is loaded, the space between the soil grains will decrease, the compressibility of the soil will descrease. The soil will become stiffer. The relation between loading and deformation is non-lineair. | 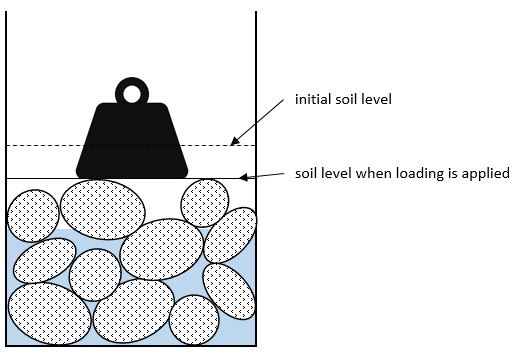 |
| When soil is unloaded, the soil will not return to it’s original position. Soil behaves plastic. | 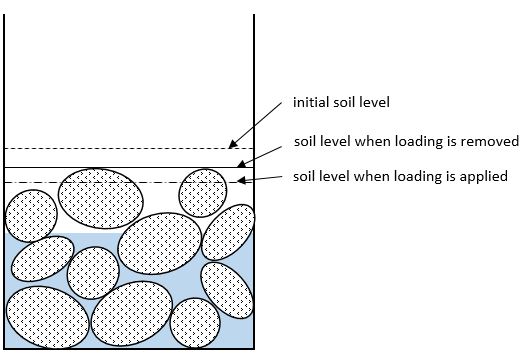 |
The relation between load and deformation in soil is given by Terzaghi’s law.
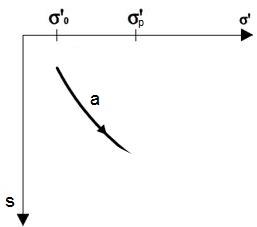
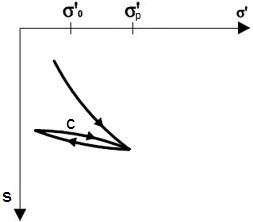
| When soil is loaded, the settlement s is determined as (curve a):
|

|
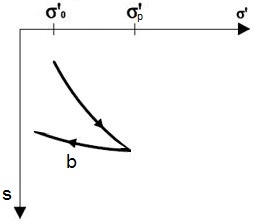
| When soil is unloaded, the settlement
|

|
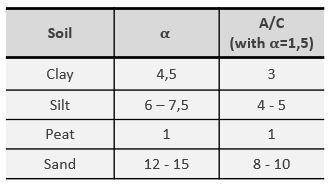
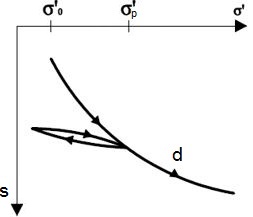
| When the soil is loaded again, it will react stiff when the stress From the moment the stress This is called overconsolidated soil. The settlement The ratio |
  |